findmyvef.blogg.se - Calculate composite functions

More Composite Functions Lessons and Learning Resources Functions Learning Material Tutorial ID There are 9 lessons in this physics tutorial covering Composite Functions, you can access all the lessons from this tutorial below. You have reached the end of Math lesson 16.4.9 Range of a Composite Function. It must be positive, so, we have R = (0, + ∞). X - 1 / x + 2 > 0 The sets of allowed values in the argument correspond to the domain of f ◦ g (x) found in (a). In this way, the domain set obtained from the common elements of the two individual domains above is

In symbols, we write Z = (-∞, -2) ⋃ (-2, + ∞) where ' ⋃' is the symbol used to describe the union of two sets, which we are going to discuss in the next chapter. Hence, the domain Z of this function includes two intervals: (-∞, -2) and (-2, + ∞). Y = (-∞, 1).Īs for the domain of the outer function f(x), it is clear that all values except x = -2 are allowed, as for this value the denominator becomes zero (it gives a not determined result). Thus, since the argument of a logarithm cannot be zero or negative, we solve the inequality 1 - x > 0 for the domain of the inner function g(x).

First, we identify the domain of the two individual functions then we find the domain of f ◦ g by taking the common elements of these two domains, as described in the previous paragraph.

Therefore, the domain of fg(x) is x ∈ R and the range is fg(x) ≥ 3.įg(x) = 1 has no real solution because it falls outside the range of fg(x) which is fg(x) ≥ 3. However, the function has real solutions for all values of x. Since the vertex is the lowest point of the curve, this means that any y values below 3 are not real solutions for fg(x) = (2x+1) 2 + 3. The x value comes from setting 2x + 1 = 0 and finding x = -½, and the y value comes from the 3 at the end of the expression. Therefore, we must find the vertex, which is the lowest point of the curve, to establish which values fg(x) holds for. Since this equation is quadratic, the graph must be a parabola which is a u-shaped curve. To find the range of fg(x) we must imagine what the graph will look like. The reason for this is that fg(x) = 1 is outside the range of fg(x).

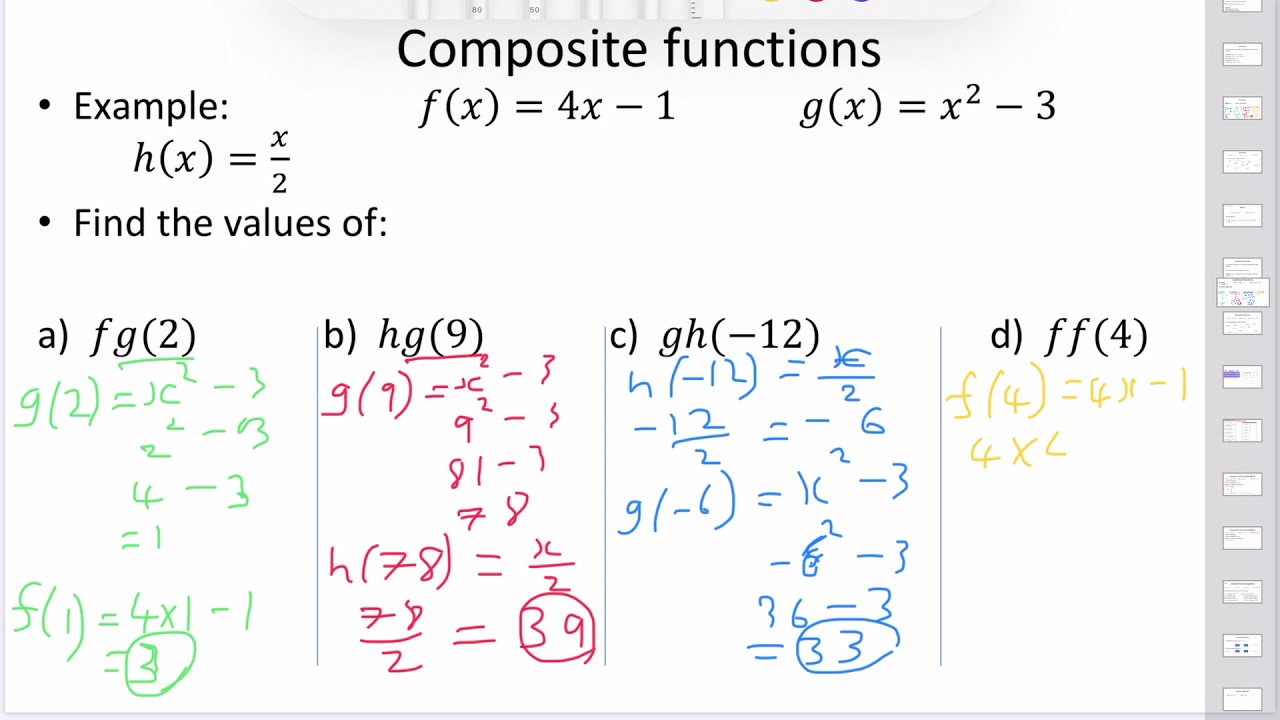
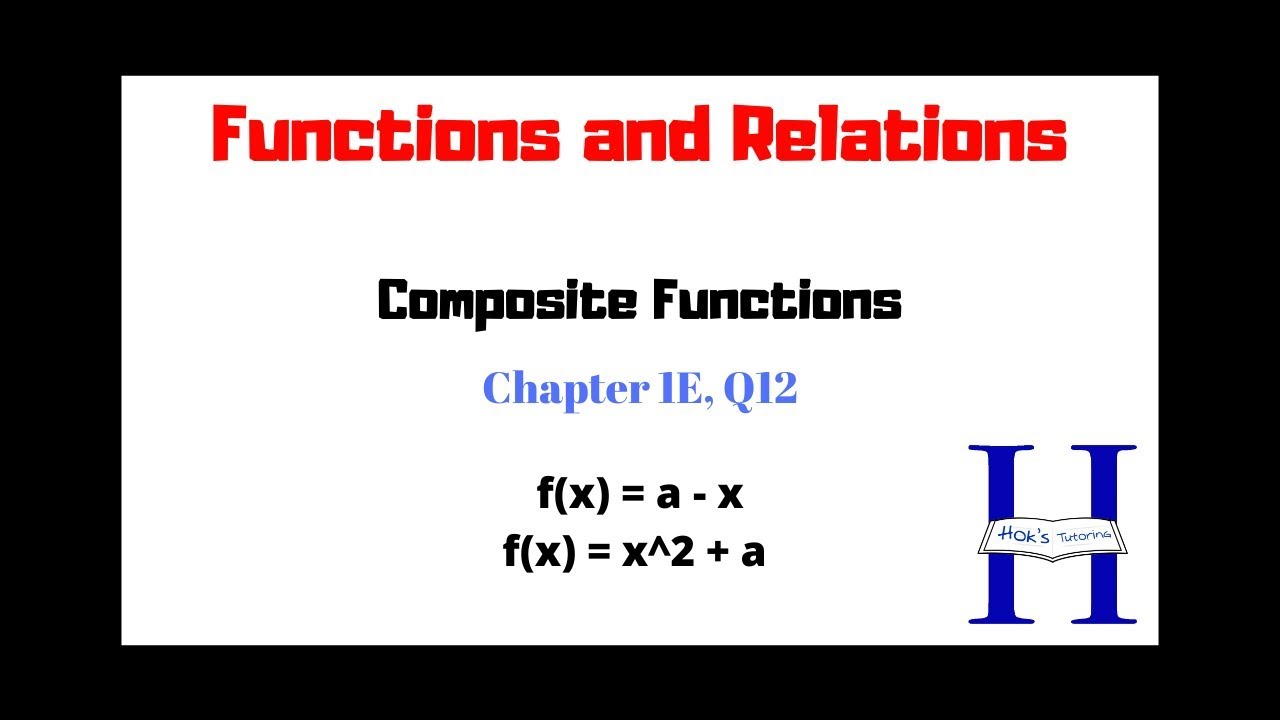
The next step we would usually take is to square root the number on the right hand side of the equation but we cannot square root a negative number without giving a complex solution, and so fg(x) does not have a real solution. If we follow the same method as before we will find that fg(x) = 1 has no real solution: Like in Q1) we must find the function fg(x) before we solve it: Q2) Find fg(x) and explain why fg(x) = 1 has no real solution. We will now use a different example from above to demonstrate this point. These values determine whether a composite function will solve for a particular value, and so it is important to find the domain and range. This result is completely different from our result of fg(x) = (x+5) 3Īnother point to consider when solving composite functions is the array of values for which the function holds i.e. For instance, from using f(x) and g(x) from our example above, we can calculate gf(x) below: One thing to note when calculating composite functions is that fg(x) is unlikely to be the same as gf(x). For example, if we apply the function f to f( x), we have ff( x) or f 2( x). It is also possible to form a composite function by applying the same function twice.

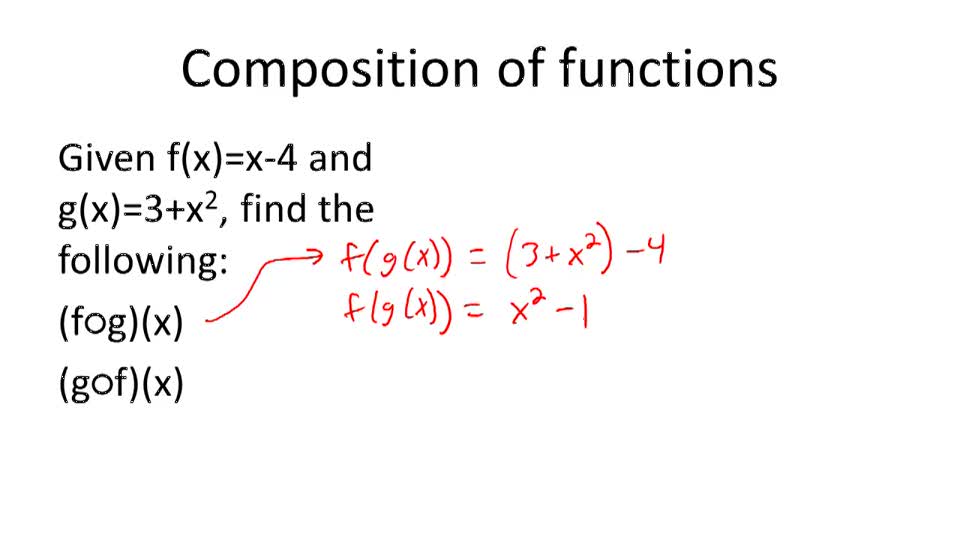
We can now use the above function to rearrange and solve for fg(x) = 27. We can now replace 'x' in f(x) = x 3 with 'x+5': In order to do this, we can break down the function in the following way:Īs you can see, we have broken down fg(x) into a function of g within a function of f. (Note: composite functions may have a different notation such as (f ○ g)(x))įirstly, we must find the composite function fg(x) in terms of x before we solve it. Composite functions can be thought of as 'functions within functions'.